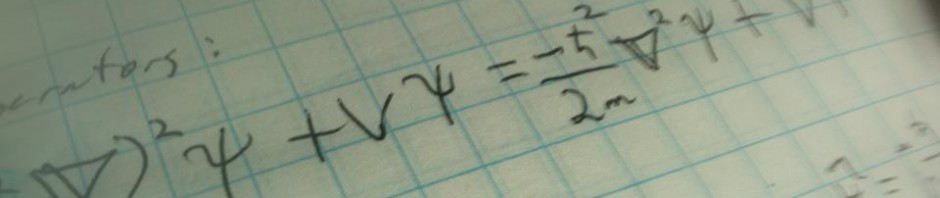Dirac “bra-ket” Notation

Hilbert Space: an abstract vector space, real or complex, of either finite or infinite dimension, in which an inner product is defined. Hilbert spaces are also complete, meaning that any point which can be constructed from elements of the Hilbert space via a Cauchy sequence is itself part of the Hilbert space. A closed linear subspace of a Hilbert space is itself a Hilbert space.

In quantum mechanics, the state of a system is generally represented as a vector in an abstract state or configuration space known as a Hilbert Space (denoted by $\mathscr{H}$ ). The evolution of the system over time is denoted by the precession of the state vector through the Hilbert space. In the traditional Schrödinger formulation, this state is a complex wave function, which can be computationally and notationally unwieldy to work with. This led P.A.M. Dirac in 1939 to introduce a new shorthand notation for representing these state vectors and calculations involving them.

Conjugate Transpose (or Hermitian Conjugate): formed from an m-by-n matrix by taking the transpose of the matrix (forming an n-by-m matrix), then taking the complex conjugate of each entry (negating their imaginary components).

If Ψ corresponds to a state vector in Hilbert space, in Dirac notation it is written as a “ket” vector with the form |Ψ>. Its conjugate transpose, or Hermitian conjugate, (which, if Ψ is simply a complex number, is the complex conjugate Ψ*) is written as a “bra” vector <Ψ|.

In general, a quantum state is regarded as a superposition of vectors (in a given basis) with different coordinate coefficients for each basis component. Depending upon the system, the number of dimensions of the basis set can be finite or infinite. In either case, the state can be thought of as a column vector:

$\mid\psi\rangle = \displaystyle\sum\limits_{i=0}^n c_i \mid i \rangle = \begin{bmatrix} c_0\\c_1\\c_2\\...\\c_n\end{bmatrix}$
where |i> represents the unit vectors of the basis set.

Similarly, the dual bra vector for this state, the Hermitian conjugate, can be represented as a row vector:

$\langle\psi\mid = \displaystyle\sum\limits_{i=0}^n \langle i\mid c^*_i = \begin{bmatrix} c^*_0 & c^*_1 & c^*_2 & ... & c^*_n\end{bmatrix}$

Multiplication of a row vector with a column vector is simply the inner product of the two vectors, resulting in a complex number:

$\langle\phi\mid\psi\rangle=c$

Multiplication of a column vector with a row vector is the outer product, and results in a matrix of complex numbers which can be interpreted as an operator (see the discussion of operators below):

$\mid\phi\rangle\langle\psi\mid=\hat{A}$

The inner product of two state vectors denoted as as <Φ|Ψ> can be interpreted as the projection of the Ψ vector onto Φ, or, specifically in quantum theory, the probability amplitude for the state Ψ to collapse into state Φ. This fact can be used to represent any state vector in terms of any arbitrary basis set:

$\psi (x)=\langle x\mid\psi\rangle$

Operators

A linear operator, which acting on a state in Hilbert space $\mathscr{H}$ results in another state in $\mathscr{H}$ , can be applied to a ket to generate a new ket consisting of a number (which can be complex) and the original ket as follows:

$\hat{A}\mid\psi\rangle = (A\mid\psi\rangle)$

Similarly, an operator can act on a bra vector from the right to generate a new bra vector, which obeys the following relationship:

$(\langle\phi\mid A) \mid\psi\rangle = \langle\phi\mid (A\mid\psi\rangle)$

This can be written more succinctly as $\langle\phi\mid\hat{A}\mid\psi\rangle$ .

If an operator is flanked by bra and ket operators for the same state vector, the result is a number representing the expectation value of the observable:

$\langle\psi\mid\hat{A}\mid\psi\rangle = \langle A\rangle$

Properties

Distributive Property

$\langle\phi\mid\left(c_1\mid\psi_1\rangle+c_2\mid\psi_2\rangle\right)=c_1\langle\phi\mid\psi_1\rangle+c_2\langle\phi\mid\psi_2\rangle$
$\left(c_1\langle\phi_1\mid + c_2\langle\phi_2\mid\right)\mid\psi\rangle=c_1\langle\phi_1\mid\psi\rangle+c_2\langle\phi_2\mid\psi\rangle$

Associative Property

For linear operators, the following relations hold:

$\langle\psi\mid(\hat{A}\mid\psi\rangle)=(\langle\psi\mid\hat{A})\mid\phi\rangle$
$(\hat{A}\mid\psi\rangle)\langle\phi\mid=\hat{A}(\mid\psi\rangle\langle\phi\mid )$

Conjugation Rules

The rules for Hermitian conjugation (denoted by the dagger $\dagger$ ) are as follows:

$\mid\psi\rangle^\dagger=\langle\psi\mid$
$\langle\psi\mid^\dagger=\mid\psi\rangle$
$c^\dagger=c^*$
$(x^\dagger)^\dagger=x$ (where x can be anything – operator, bra, ket, number)
$(c_1\mid\psi_1\rangle+c_2\mid\psi_2\rangle)^\dagger=c_1^*\langle\psi_1\mid+c_2^*\langle\psi_2\mid$
$\langle\phi\mid\psi\rangle^\dagger=\langle\phi\mid\psi\rangle^*=\langle\psi\mid\phi\rangle$ – remembering that the inner product of a bra and a ket is simply a complex number, and that the Hermitian conjugate of a complex number is simply its complex conjugate.
$\langle\phi\mid\hat{A}\mid\psi\rangle^*=\langle\psi\mid\hat{A}^\dagger\mid\phi\rangle$
$\langle\phi\mid\hat{A}^\dagger\hat{B}^\dagger\mid\psi\rangle^*=\langle\psi\mid\hat{B}\hat{A}\mid\phi\rangle$
$((c_1\mid\phi_1\rangle\langle\psi_1\mid)+(c_2\mid\phi_2\rangle\langle\psi_2\mid))^\dagger=(c_1^*\mid\psi_1\rangle\langle\phi_1\mid)+(c_2^*\mid\psi_2\rangle\langle\phi_2\mid)$

Commutation

In general, operators are not commutative (although they can be in some cases):

$\hat{A}\hat{B}\mid\psi\rangle\neq\hat{B}\hat{A}\mid\psi\rangle$
We measure the lack of commutativity by defining a commutator:

$[\hat{A},\hat{B}]=\hat{A}\hat{B}-\hat{B}\hat{A}$

In the construction of quantum theories from classical physics (using the procedure known as “first quantization”), commutators take the place of classical Poisson brackets. Also, by Ehrenfest’s Theorem, the change with respect to time of any observable can be calculated by taking the commutator of the corresponding operator with the Hamiltonian operator:

$\frac{\partial A}{\partial t}=[\hat{A},\hat{H}]$

Inverse Operators

A linear operator may possess an inverse which satisfies the following relationship:

$\hat{A}\hat{A}^{-1}=\hat{A}^{-1}\hat{A}=1$

In finite-dimensional spaces, inverses, if they exist, act equally on the left and the right:

$(\hat{A}\hat{B})^{-1}=\hat{B}^{-1}\hat{A}^{-1}$

This does not necessarily hold true in infinite-dimensional spaces.

Hermitian Operators

A linear operator is said to be Hermitian if it is its own adjoint (in other words, self-adjoint):

$\hat{A}^\dagger=\hat{A}$

In quantum mechanics, physical observables (such as energy or momentum) are represented by self-adjoint operators.

Unitary Operators

A linear operator is said to be unitary if

$\hat{U}^\dagger\hat{U}=\hat{U}\hat{U}^\dagger=\hat{I}$ where $\hat{I}$ is the identity operator.

Transformations such as rotation and time are represented by unitary linear operators.

Prerequisites:

Linear Algebra/Functional Analysis
Hilbert Spaces

Reading List – Books:

Reading List – Articles:

P.A.M. Dirac, “A New Notation for Quantum Mechanics”. Proceedings of the Cambridge Philosophical Society 35: pp. 416-418 (1939)

Lecture Notes, Lectures, and Presentations:

“The Mathematical Formalism of Quantum Mechanics” – lecture notes from Berkeley
Quantum Mechanics: A graduate level course – course notes by Richard Fitzpatrick, UT-Austin. Of particular interest regarding the topic at hand are the sections on ket space, bra space, operators, and the outer product.
Bra-ket notation – lecture notes by B. Zygelman, UNLV.

External References:

Bra-ket notation – Wikipedia, the free encyclopedia